Deformation of Frames Model MT 035
Deformation of Frames Model MT 035
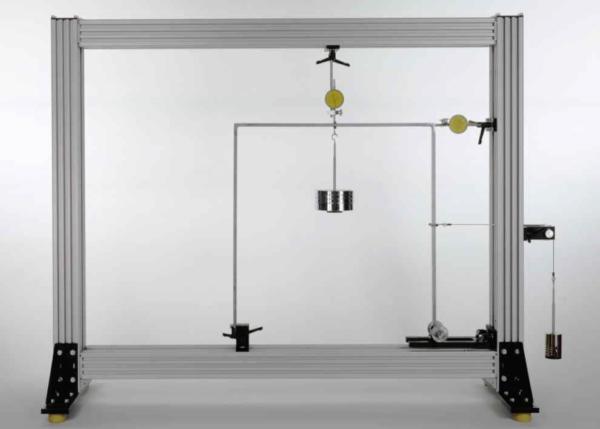
Sci-tech Deformation of Frames Model MT 035 includes a typical U-shaped frame, such as is used in the construction of halls for example. One end is clamped into place, while the other can be loosely mounted. When the non-clamped end remains free, the statically determinate frame is investigated. A roller bearing on the non-clamped end creates a statically indeterminate frame. The frame is placed under load by two sets of weights. The load application points are movable. Two dial gauges record the deformations of the frame under load.
By applying various methods (first-order elasticity theory; the principle of superposition; and the principle of virtual work), the bending moment characteristics are ascertained for a statically determinate and indeterminate frame. From these characteristic curves and a chart for integrals (coupling table) the differential equation of the bend line is formulated. From the bend line and its derivations, displacements and the support force on the movable support can be calculated.
A second, S-shaped frame can be used to show that the various methods are applicable to any kind of frame. All the component elements of the experiment are clearly laid-out and housed securely in a storage system.
| Size: | 110cm x 40cm x 100cm (LxWxH) |
| Weight: | 15 kg |
Item Description
Features
* Elastic deformation of a statically determinate or indeterminate frame under point load1
* U-shaped and S-shaped frame1
* Principle of virtual work to calculate the deformation and support reaction in a statically indeterminate system
A frame is a bent beam with rigid corners which creates a so-called structure gauge. This means that it spans a gap while at the same time creating height.
Sci-tech Deformation of Frames Model MT 035 includes a typical U-shaped frame, such as is used in the construction of halls for example. One end is clamped into place, while the other can be loosely mounted. When the non-clamped end remains free, the statically determinate frame is investigated. A roller bearing on the non-clamped end creates a statically indeterminate frame. The frame is placed under load by two sets of weights. The load application points are movable. Two dial gauges record the deformations of the frame under load.
By applying various methods (first-order elasticity theory; the principle of superposition; and the principle of virtual work), the bending moment characteristics are ascertained for a statically determinate and indeterminate frame. From these characteristic curves and a chart for integrals (coupling table) the differential equation of the bend line is formulated. From the bend line and its derivations, displacements and the support force on the movable support can be calculated.
A second, S-shaped frame can be used to show that the various methods are applicable to any kind of frame. All the component elements of the experiment are clearly laid-out and housed securely in a storage system.
The well-structured instructional material sets out the fundamentals and provides a step-by-step guide through the experiments.
See also different:
Specifications OUTPUT Waveform - Square Frequency and Amplitude Adjustment is provided using Potentiometers. One LED indicator to indicate Power Input. On-board Circuits Adder. Subtractor Integrator Differentiator. Fixed DC p [...]
